Folding

Take a simple piece of paper. Give yourself 1 minute. Crumple the paper. Notice how all of a sudden it holds itself up. How did that happen? Sketch what you have created. Pay attention to the high points and the low points. Make it into architecture. Imagine a kind of building? What happens there? How big is it? Draw some people? What is it scale? You have just transformed flatness into three-dimensional form. You have given the form a purpose. You have made something that attracts and gathers people. Name it. Draw it. Get excited about folding!

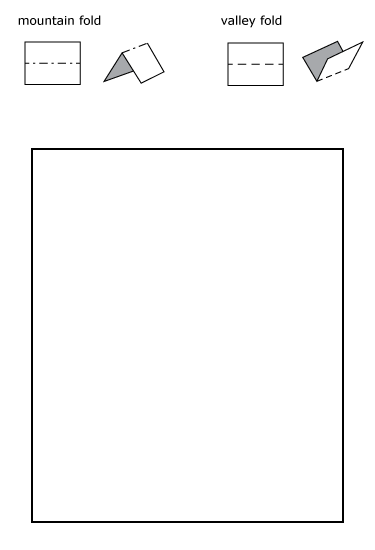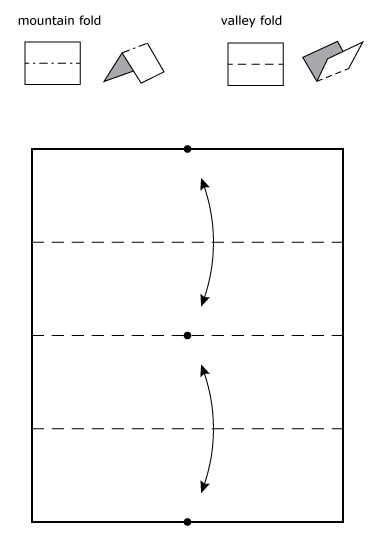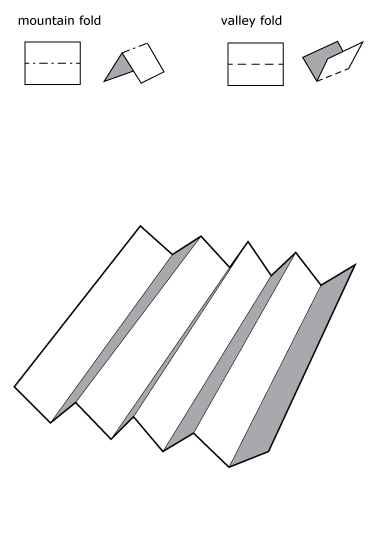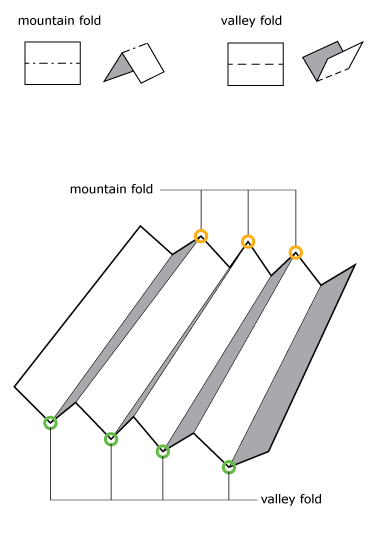
Instead of fast and furious, folding can be a geometric method for building form. The most basic types of a fold are the mountain and the valley fold. Imagine a mountain. It stands up. Mountain folds rise. Then think about a valley. Valleys are low-lying parts of the land. They are part of the land that goes down. Take one sheet of paper. Fold it in half, carefully pressing it, and then open it back up. Then fold the upper half down to the center and the lower half up to the center. Be sure to crease clearly, or press the fold each time all the way from the beginning to the end. Next, fold the bottom edge up to the top fold and open the paper. Take the top edge to the bottom fold and crease and open the paper. Fold the top edge to the top crease and the bottom edge to the bottom crease. You have created seven valley folds. TURN the PAPER OVER. From this side, you now have seven mountain folds! Mark alternate mountain creases with small Xs in the center of each alternate crease. Now fold the bottom edge up four times, each time to a marked crease. You will be making valley folds on this side. Finish with taking the top edge down to each of the four marks. You have magically divided the paper into sixteen equal sections. Alternate mountain-valley-mountain–valley and create your first paper landscape! Make a wall, abridge or a star column! Challenge yourself with a second Mountain/Valley Fold. Which fold makes a stronger bridge? You are structuring paper!

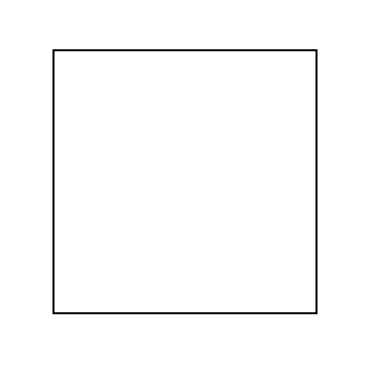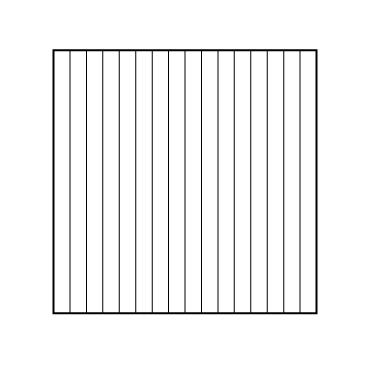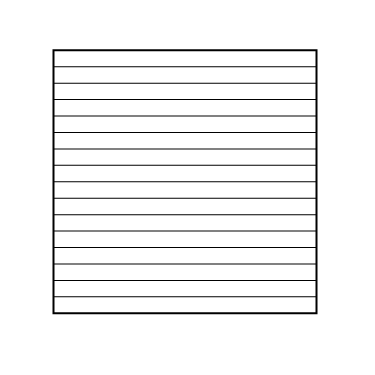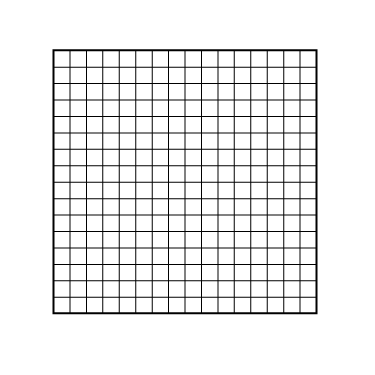
The most basic grid begins with a square sheet of paper where the sixteenth linear folds are creased parallel to the edges first vertically and then horizontally. Folding paper in a grid will take its flatness and encourage it to curve up. The grid creates the faceting or creased edges which turn the paper inward. Try a simple Square Grid. Move to folding a rectangular grid. How is the folded paper different? What forms can your folded grid paper make? You are becoming a grid folding expert!

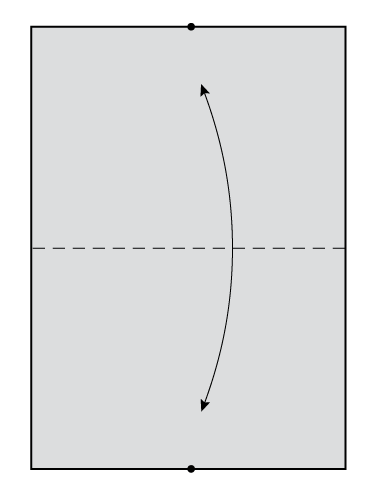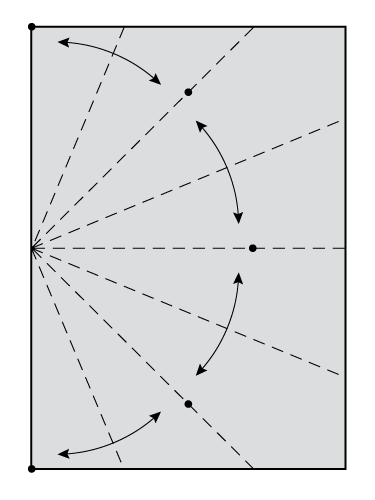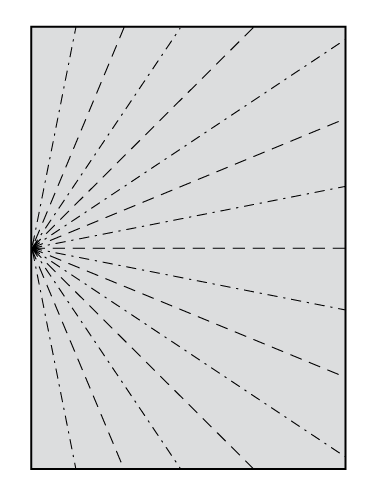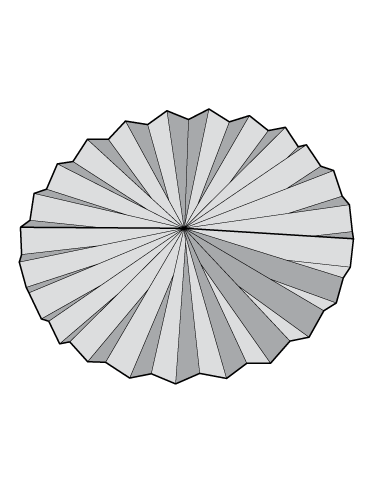
Radial folds are folds that originate from one point. Radial folds can be made as a Quarter Fold, a Half Circle fold, and a partial radial fold. The key is to be precise about the edge of the fold coming cleanly to the point as much as possible. The folds, when complete, serve as fans or can be connected on the open edges to form 3 dimensional forms. Start with a simple [Quarter Radial Fold[https://drive.google.com/open?id=1_HVt-aBpgQs4NFGYjiqLS0pShj-avpxE]. Move to a Half Circle Fold. Next, try a ¾ Circle Fold. Finally, complete a Full Circle Fold. How does the shape of the flat fold dictate its form and height? Take pictures of the folds and then the forms that you created!

Be radically radial radiant!

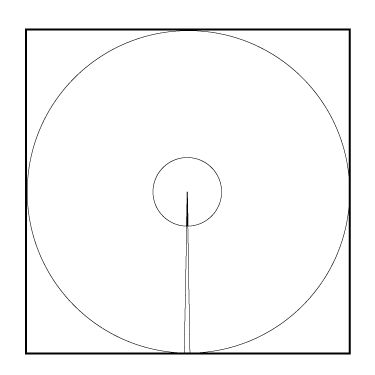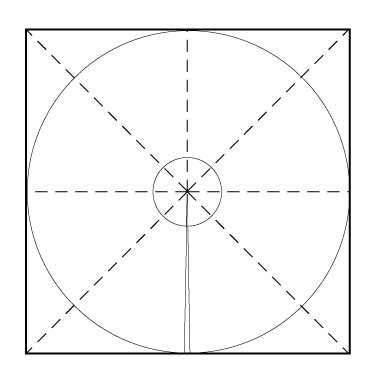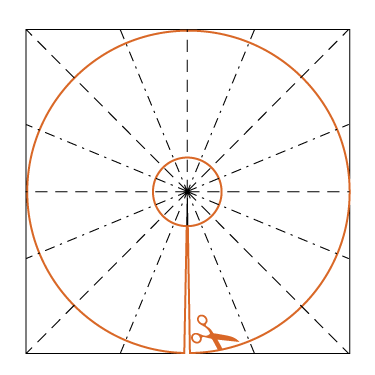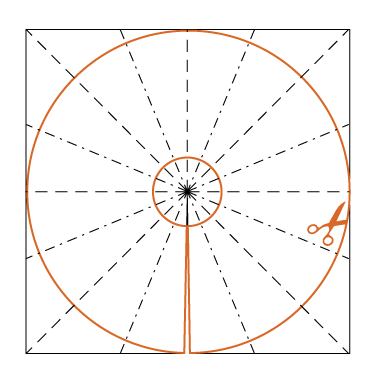
Start with a new sheet of square paper. Make the top edge meet the bottom edge, folding the paper in half. Next fold the lower right side of the paper to the center crease and crease. Mirror this by folding the top side to the center. Open the paper. This time, fold the lower right edge of the paper to the last top fold. At the same time, take the top right side of the paper to the last bottom fold. Next fold the lower right to the last bottom fold. Fold the upper right to the last top fold. You should have seven valley folds and eight equal angles. TURN the Paper Over! Lightly mark each alternate mountain crease with an x.Now fold the right lower side of the paper to all of the x marks, opening the paper back up each time. Finish by folding the top right side to all of the x marks from the top. You now have 16 equal angles. Use alternate mountain and valley folds to create your new landscape. You can also create a rotational diagonal, rotational fold. Try this complete circle fold.

Rotate! Radiate! Fold! Form!

Another adventure is to start with a square sheet of paper. Turn the paper like a diamond in front of you. Fold the right corner over to the left corner. Then fold the top corner down to the bottom. Now fold the top to the middle and open. Bring the bottom corner to the middle and open. Now using the folding methods of activity 1 fold linear valley folds and turn the paper over and fold the mountain folds. If you fold the entire diamond in half, what happens to the form?!!! Try some variations of Square folding here and here.Amazing new forms are created!

Now that you are becoming a folding expert, try experimenting with diverse diagonal translation folds. Observe the differences in Translation Fold 1, Translation Fold 2, Translation Fold 3 and Translational Fold 4. Translating a pattern uses repetition as well as symmetry as the folding lines repeat.

Now that you are an expert at folding, you can fold larger sheets into an endless array of forms.

• Can a piece of paper be folded in halves 12 times?
  • Yes
  • No
• Mountain Folds higher than valley folds.
  • True
  • false
• Rotational Folds radiate from a point.
  • True
  • False
• Translationational symmetry folds repeat horizontally only.
  • True
  • False
• Shelters can be made from folded water proff papers.
  • True
  • False